In 1593, German mathematician Christopher Clavius made a small mark that would change mathematics forever.
In a sine table in his treatise on the astrolabe, Astrolabium, he indicated the fractionation of a whole number by writing what has come to be regarded as the very first use of the decimal point.
There is, however, just one problem. According to new painstaking research by historian Glen Van Brummelen of Trinity Western University, this wasn’t, in fact, its first instance. After poring painstakingly over historical documents, Van Brummelen found much earlier instances of the tiny notation.
In the 1440s, a young Italian merchant by the name of Giovanni Bianchini penned several astronomical works that made extensive and increasingly refined use of decimal points.
Van Brummelen convincingly argues Clavius merely copied the decimal point from Bianchini, who should be credited for his mathematical innovation.
Bianchini, born in around 1410, was a Venetian merchant who had probably been trained in arithmetic and algebra. Sometime in the 1430s, he was retained by the House of Este as an administrator of the estate; it was in this role that he penned his astronomical treatises, probably because his role involved astrological prediction for his noble employers.
He wrote five major works between 1440 and 1460: Tabulae astronomicae, on the motions of the planets; the Tabulae primi mobilis A, on spherical astronomy and mathematical astrology; the Flores Almagesti, his masterwork on mathematical astronomy; the Tabulae primi mobilis B, a rewritten version of the Tabulae primi mobilis A; and the Tabulae eclypsium, on the prediction of eclipses.
At that time in Europe, astronomy was performed using sexagesimal arithmetic; a system that uses 60 as its base the way our modern decimal system is based on units of 10. Though rarely used today, sexagesimal arithmetic has a lasting legacy in today’s timekeeping.
Bianchini’s Flores Almagesti starts with two chapters; the first on arithmetic, and the second on algebra. Giving such focus to these two topics might seem out of place in an astronomical work, but they set the groundwork for Bianchini’s translation of mathematical astronomy from sexagesimal to a type of decimal arithmetic of his own devising.
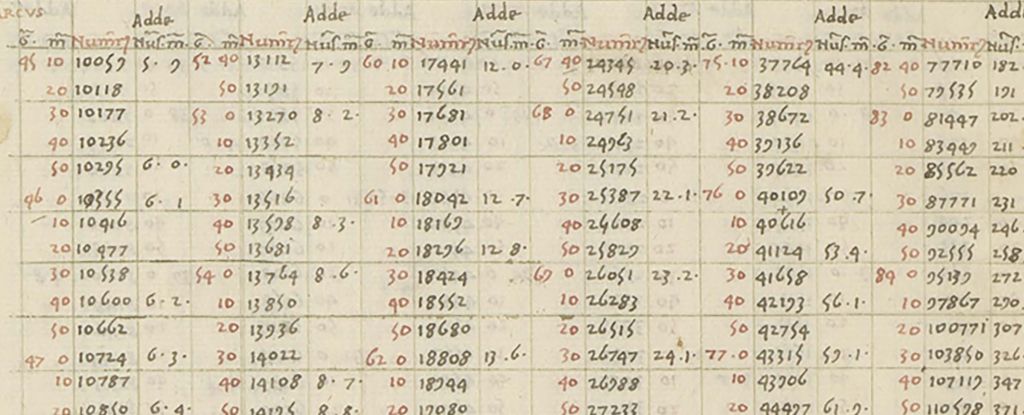
Obviously Bianchini’s system didn’t really take; the sexagesimal system was still being used, much later, for astronomical calculations. But in his discussion of trigonometric functions in Tabulae primi mobilis B, Bianchini made his literal mark. Describing the use of interpolation to find the sine and cosine of an arc, he casually drops the number 10.4, and explains how to multiply it by 8, giving the answer 83.
The product of 10.4 and 8 is 83.2, but Bianchini’s working shows that he is using the decimal point, and understanding it, the same way we use it and understand it.
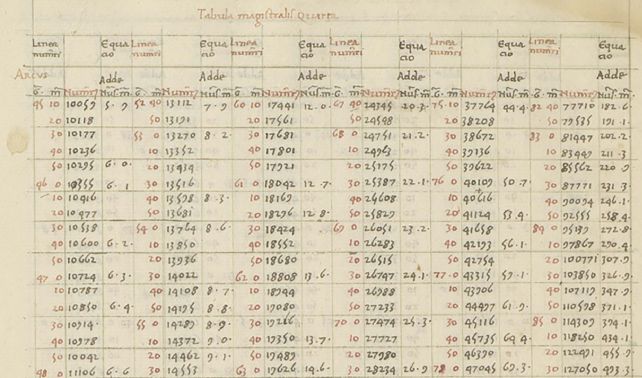
Then, more dots show up in sine tables in the same work, in the interpolation column. This is exactly how the decimal point shows up in Clavius’ Astrolabium, used in precisely the same way to perform the same function. And, tellingly, Clavius never uses it again, that we know of.
As someone who was a learned and respected scholar of astronomy, it’s likely that Clavius knew of the work of Bianchini. This suggests an explanation for the decimal point’s single appearance in Clavius’ writings, Van Brummelen says.
“He had access to Bianchini’s sine table (or to someone who himself had borrowed from Bianchini), and he copied the structure of that table in his own work,” the historian explains in his paper.
“He recognized the usefulness of decimal fractional notation in the context of interpolation within a numerical table; but it was not his idea, and likely he had not invested significant effort himself to explore the use of the notation more fully. As a result, the origin of decimal fractional notation and the decimal point in Europe remained obscure until now.”
It’s such a mind-blowing discovery, and suggests that Bianchini’s role in the complex and fascinating development of modern mathematics may have been somewhat overlooked. Perhaps there are more such discoveries out there, lurking in the pages of ancient books.
Van Brummelen’s findings have been detailed extensively in the journal Historia Mathematica.